NavList:

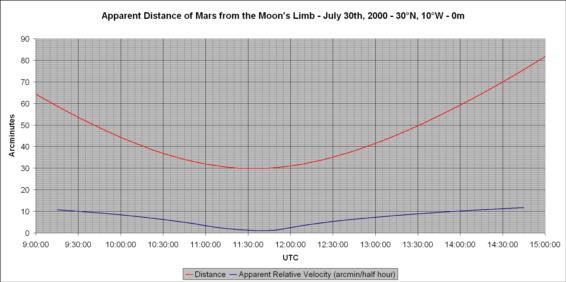
If I correctly understand what Antoine is describing (and there is a very real posibility that I don't), then I have done some experimenting with this phenomenon in the very recent past. I was trying to understand why it is not recommended to shoot lunars when the distance is very short. I created a graph which showed the lunar distance along with the apparent relative velocity between the two bodies. Unfortunately, I cannot find that graph on my computer now. So, I made the attached graph using the example Antoine gave in his post about the occultation of Mars by the moon. In this graph, the apparent distance of Mars from the moon's limb is shown by the red curve. The apparent velocity between the two bodies is shown by the blue curve. You can see that the relative velocity decreases (and increases again) in a non-linear fashion in proportion to the distance. I suppose that the velocity would decrease to zero at some particular instant, although that is not shown on the graph due to the curves being smoothed representations of values calculated every half hour.

To create the graph, I used MICA to calculate the topocentric LHA and Dec. at the selected location for each half hour, three hours on either side of 1200 hrs. UTC. I then used the formula acos(sin(Dec1)·sin(Dec2)+cos(Dec1)·cos(Dec2)·cos(LHA2-LHA1)) to calculate the apparent distance in Excel. Finally, I subtracted the SD of the moon (to approximate the distance from the limb) and calculated the difference in distance between each half hour. What the graph does not [entirely] explain is the reason(s) for the non-linear change in velocity. At any rate, I suppose most, if not all of the factors are represented therein.

Am I way off the mark here? I'd be most interested in anyone's comments on this. Thanks!

Sincerely,

Sean C.

File: