NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
Re: Advancing the LOP
From: Gary LaPook
Date: 2015 Apr 28, 07:43 +0000
NavList Message
From: Robert Bernecky <NoReply_Bernecky@fer3.com>
To: garylapook@pacbell.net
Sent: Monday, April 27, 2015 11:16 AM
Subject: [NavList] Re: Advancing the LOP
Attached File:

(f1-Advancing-the-LOP-Problem.pdf: Open and save or View online)
From: Gary LaPook
Date: 2015 Apr 28, 07:43 +0000
Since you brought up the symmedian point, here are some links to when we discussed this back in December 2010.
gl
From: Robert Bernecky <NoReply_Bernecky@fer3.com>
To: garylapook@pacbell.net
Sent: Monday, April 27, 2015 11:16 AM
Subject: [NavList] Re: Advancing the LOP
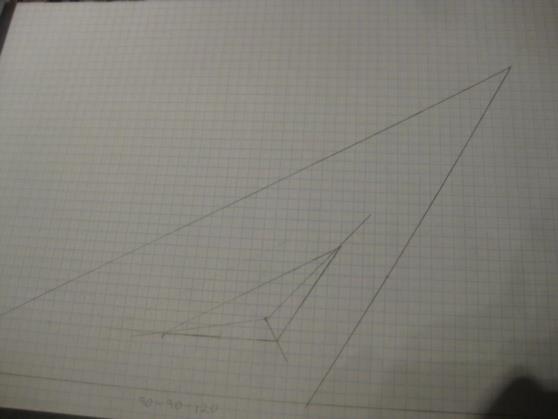
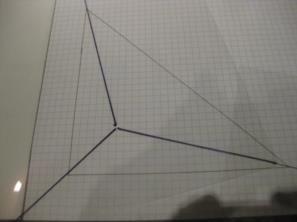
Following up on Herbert's post, Herbert has a nice geometric proof that the symmedian point (Gergonne point) (see http://math.wikia.com/wiki/Inscribed_circle) is the "least-squares" position. As shown in the attachment, it is found by (1) drawing the in-circle, (2) marking the points where the circle is tangent to the triangle's sides, (3) drawing a line from each vertex of the triangle to the opposite sides tangent point, (4) noting the intersection of these lines is the symmedian point.
Attached File:

(f1-Advancing-the-LOP-Problem.pdf: Open and save or View online)