NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
From: Robin Stuart
Date: 2015 Feb 21, 14:17 -0800
I have been playing around with some new geodetic plotting functionality introduced in the program Mathematica and have used it to produce some jpeg’s that illustrate a point relevant to threads from 2006
https://NavList.net/m2.aspx/Advancing-position-circle-was-Positionfrom-crossing-two-Bergman-jun-2006-w30924
https://NavList.net/m2.aspx/Advancing-position-circle-PeterFogg-jun-2006-w30890
https://NavList.net/m2.aspx/Advancing-position-circles-Huxtable-vs-Zevering-Bailey-sep-2006-g1311
https://NavList.net/m2.aspx/Advancing-COPs-Revisited-Zevering-may-2010-g12946
https://NavList.net/m2.aspx/Advancing-position-circles-Huxtable-vs-Zevering-Bergman-mar-2010-g12126
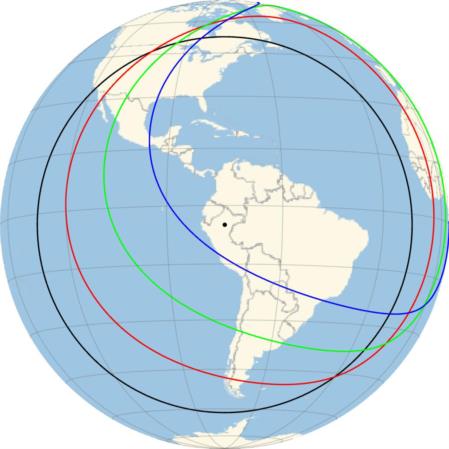
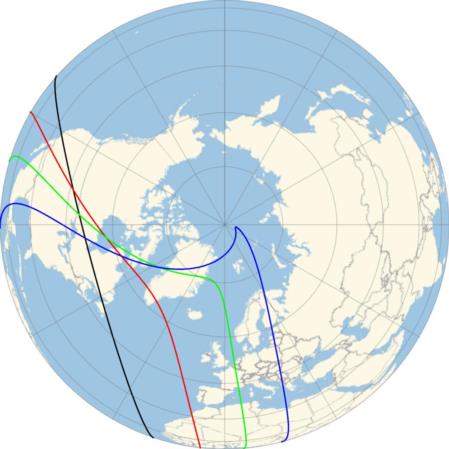
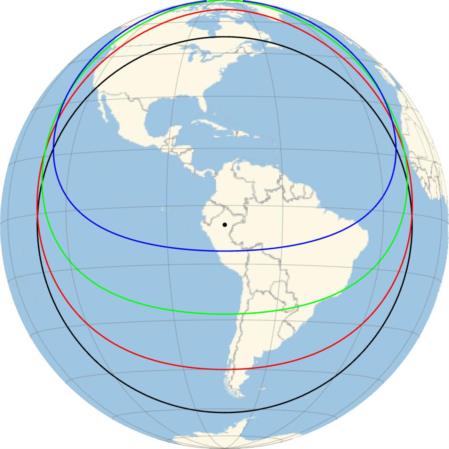
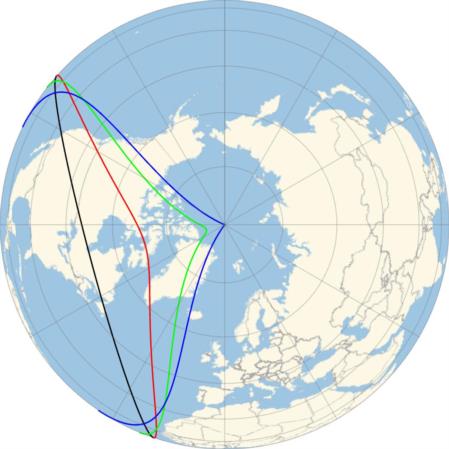
These concerned advancing a circle of position (CoP) and finding its intersection with a second later CoP to obtain a fix. To advance the first CoP each point needs to be moved along a rhumbline of specified distance and course. If a rhumbline is drawn far enough it will form a spiral that eventually reaches the North or South pole. (Some plots of this can be found here https://en.wikipedia.org/wiki/Rhumb_line ). Each point on the first CoP is advanced along a distinct spiral path which means that after a finite displacement the original CoP is a circle no longer. This point was made by the late George Huxtable on Navlist and in print (The Journal of Navigation, 59 (2006) 521). It follows that any attempt to get running fix by simply crossing 2 circles is fatally flawed. This point provoked a fair amount of confusion and intense debate back in 2006 and again in 2010.
The attached jpegs show an initial CoP with geographic position (GP) at 4 55.4’S 74 59.7’W and with radius of 56 42.3’. This CoP is shown in black. In the first jpeg the red, green and blue curves are the result of advancing each point on the original CoP by 1000nm, 2000nm and 3000nm respectively on a rhumbline course bearing 45. They are viewed from a point above the GP. The second jpeg shows the same set of curves viewed from above the North pole. The next set of two jpegs is the same as the first two except that the course is due North. Note that points on the blue curve have reached the pole from where they cannot escape as if from a black hole!
Robin Stuart