NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
From: Robin Stuart
Date: 2015 Nov 24, 09:23 -0800
https://NavList.net/m2.aspx/Interpolation-Meridional-Part-Table-Huxtable-mar-2009-g7774
The inconsistencies are summarized as follows
- Bowditch calculates Meridional Parts to a very high level of accuracy in terms of geographical miles (1 minute of longitude) and the parameters of the ellipsoidal WGS72 datum. These are used in Mercator Sailing calculations as if they were in nautical miles (roughly 1 minute of latitude).
- For the calculation of distance along meridional arcs Bowditch 2002 uses the latitude difference in minutes of arc for nautical miles. This is only correct assuming a spherical model of the Earth. The formula for the length of a meridional arc on an ellipsoidal Earth can be expressed in terms of Elliptic Integrals of the second or third kind and is found in https://en.wikipedia.org/wiki/Meridian_arc
The general procedures for Mercator sailings on an ellipsoid Earth are given in the book “The Geometry of Navigation” by Roy Williams and allows the errors introduced by these inconsistent assumptions regarding the figure of the Earth to be quantified.
Because 1 geographical mile = 1.00097 nautical miles, the first bullet above introduces a constant error of 0.1%.
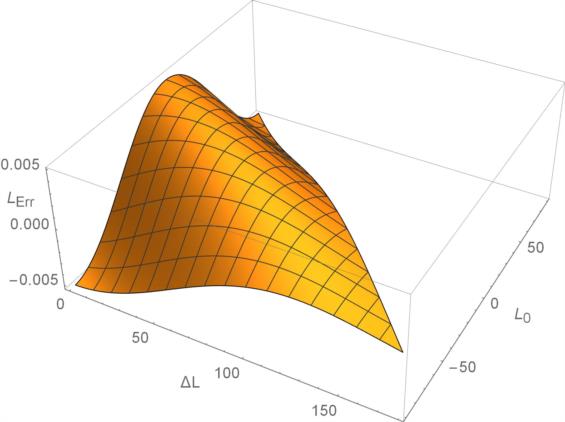
Errors arising from the second bullet depend on the initial latitude and length of the meridional arc traversed. In absolute terms the maximum possible error comes out to 16.7 nautical miles which occurs for a meridional arc running from roughly 45° S to 45° N. However it represents only 0.3% of the total distance travelled. The maximum possible percentage error is around 0.5%.
The attached figure shows the fractional error as a function of initial latitude (L0) and degrees of latitude traversed (ΔL) northward from the initial point.
So for the examples in Bowditch 2002 section 2416 how different are the answers if computed using a fully consistent methods are applied to an ellipsoidal Earth (WGS72)?
Example 1: A ship at lat 32°14.7’N, long 66°28.8’W is to head for a point near Chesapeake Light,36°58.7’N. long 75°42.2’W.
Bowditch gives
Course = 301.8°
Distance = 538.2 miles
Full ellipsoidal WGS72 result is
Course = 301.8°
Distance = 537.3 miles
Example 2: A ship at lat 75°31.7’N, long 79°08.7’W, in Baffin Bay, steams 263.5miles on course 155°.
Bowditch gives
L2 =71°32.9'
λ2=072°34.1’
Full ellipsoidal WGS72 result is
L2 =71°33.9’
λ2=072°35.6’
The two positions differ by 1.15 nautical miles.
In order to perform the full ellipsoidal calculation Bowditch would presumably need to include tables of Meridional Arcs, similar to the tables of Meridional Parts already present, as well as their inverse. Given the magnitude of the errors introduced this is probably not warranted in a book with the word “Practical” in its title.
Robin Stuart