NavList:

William Chauvenet developed a method for clearing lunars (working longitude by lunar distances, angles between the Moon and other celestial bodies) at the tail end of the era when lunars were in practical use at sea. His method was arcane and exotic, and there's no evidence that it was ever used by practical navigators. It would probably be forgotten today had it not been published as an epilogue to the technique of lunars in editions of the radically revised "Bowditch" at the end of the 19th century (and secondarily, if the inexpensive Dover edition of Chauvenet's "Spherical and Practical Astronomy" had not become a fetish object among "Baby Boom" era astronomy enthusiasts).

Chauvenet referred to his own method as "the greatest thing since sliced bread" (not literally, since the invention of sliced bread was actually 75 years in the future when Chauvenet was working! ...but you get what I mean). I was reminded of this by Dave Walden's recent post. Chauvenet wrote:

In the mean time I have found that something could be done
towards perfecting the common methods without changing the
form of the ephemeris, and without introducing any processes
of a kind not familiar to practical men. The method I have
proposed is hardly more laborious than the simplest of those
in common use, while it attains to that extreme precision which
is required when we wish to get from our observations all that
they are capable of giving.

This is a boast. To be fair, it was a normal style of boast when any "new" method of working lunars was trotted out in this period. All too often... "My new method" is the "most accurate" and "simplest to work". They all were! In this half-century, c.1825-1875, numerous amateur mathematicians as well as marginal professional mathematicians believed they could "fix" the "wrong methods" of lunars, as they perceived them, and finally make lunars practical.

Chauvenet introduces his new method by discussing a method proposed by Friedrich Bessel, another mathematician who thought he could make lunars practical by introducing a new model clearing method. It did not succeed. Chauvenet wrote:

This failure is to be ascribed chiefly
to the nature of Besset’s computation, which, besides being
considerably longer than those in common use, required more
skill in the management of trigonometric functions than was
generally possessed by navigators. As delivered by its author,
the method seemed simple enough to the mathematician
accustomed to considering the varying signs of his functions,
but when it was reduced to a set of practical rules, dispensing
with a consideration of those signs, it became embarrassing by
the multiplicity of its cases. But the solution is the only complete
and perfect one that has been given, and cannot be wholly laid
aside. It is to be hoped that an ephemeris adapted to this
method may again be established, if not for the use of all
navigators, yet for those (and they are not a few) who possess
the requisite skill, and who set a value upon precise results.

Chauvenet, as a mathematician, is offering a mathematician's unhistorical theory here. I suggest that Bessel's method, like Chauvenet's decades later, failed in the marketplace because it solved no significant problem, and other excellent tools already existed, well-known to that dwindling community of lunarians in the later decades of the 19th century. That's the simplest explanation. No one needed it.

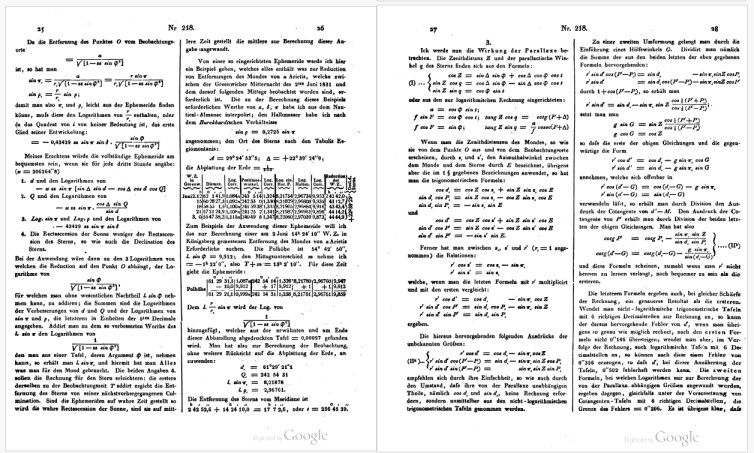
Now and then in the past few decades, lunarians and other fans historical navigational computations have asked if anyone could supply Bessel's original article. The usual internet searches did not locate it. Quite possibly someone has posted it or provided a link to it for the NavList community in some earlier year, and I somehow missed it. If that's the case, I don't suppose there's any harm in another copy. I realized a couple of years ago that articles outside the English language are not well indexed in English-language results by Google and other search engines. The German language Wikipedia article on the Astronomische Nachrichten provided links to archives of historical volumes, and that made it simple. Attached here is Bessel's original 1832 "orphan" method for clearing lunars (German language). I call it an "orphan" because it has uncertain parentage, and also, like an orphan in an old tragedy or a cartoon, it is "unloved".

Frank Reed
Clockwork Mapping / ReedNavigation.com
Conanicut Island USA

File: