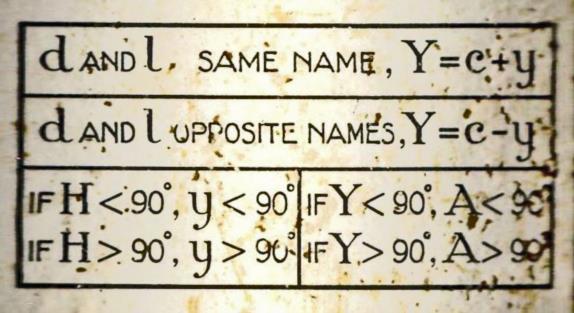
Special Cases
I took a look at the method described for calculating azimuth when the declination is less than 20’
https://navlist.net/m2.aspx/Comments-BygraveMHR1-calculations-LaPook-aug-2016-g36156 and found that it works extremely well. Kudos to Gary. A plot of the error over the full range of possible latitudes and LHA’s reveals that it never exceeds 20’. The method involves setting “y” (Bygrave nomenclature) equal to the declination and must be used with the condition that y is greater than 90°when H is greater than 90°.
Evaluating other equations on the Bygrave/MHR1
1) Set pointer I (S) to 0° and set pointer II (L) to 6° by moving the cotangent cylinder.
2) Keeping the cotangent cylinder fixed move pointer II to 15° ( = 45°- (75°-45°) = 90°- 75° ).
3) Read the required value from pointer I (66°54.3’)
When both δ and L are less than 45° the operations change. Evaluating cos(LHA) = -tan δ tan L for δ = 35°S and L = 6°N gives LHA = 85°46.8’.
1) Set pointer I (S) to 0° and set pointer II (L) to 6° by moving the cotangent cylinder.
2) Keeping the cotangent cylinder fixed move pointer II to 45°.
3) Move the cotangent cylinder to set pointer II to 35°.
4) Keeping the cotangent cylinder fixed move pointer II to 45°.
5) Read the required value from pointer I (85°46.8’).
It seems likely that operations could be found that would allow cylindrical Bygraves to evaluate any and all of Napier’s rules for spherical right triangles and quadrantal triangles.
I attach enhanced versions of images of the Bygrave instructions and corrections posted previously,
Robin Stuart
Attached File: 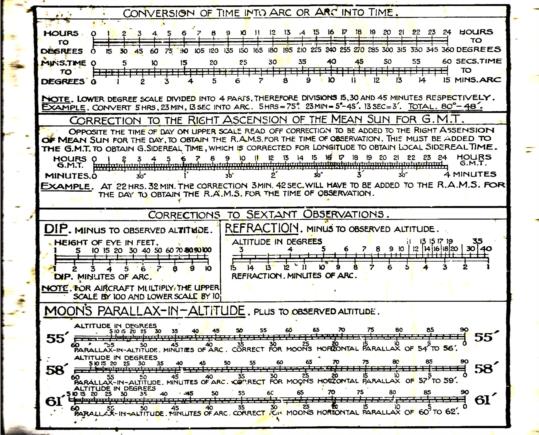
 Attached File:
Attached File: 
 Attached File:
Attached File: 
 Attached File:
Attached File: