NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
From: Frank Reed
Date: 2019 Aug 4, 19:53 -0700
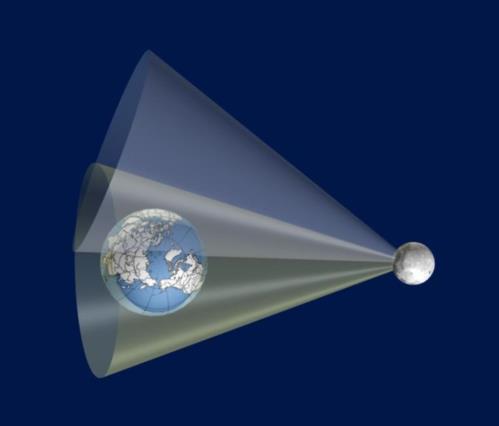
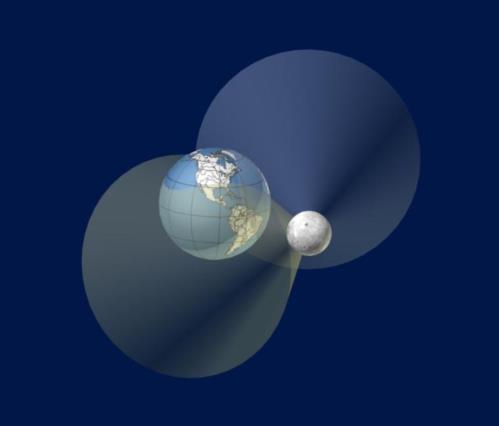
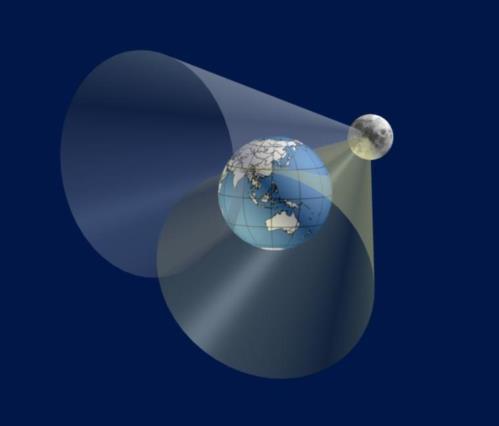
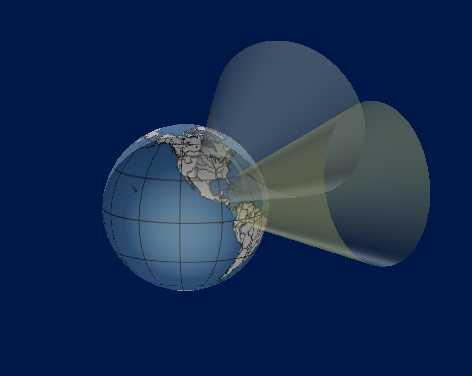
I didn't feel I had time to dig for old posts, so I'm adding these 3D illustrations that I prepared years ago just to get you started. Take a close look at cop1,2,3 to get a sense of how cones of position from lunar arcs ("altitudes" measured off the Moon's distant horizon) would eventually intersect the Earth as nearly flat planes. In this illustration, the scale is compressed. In a real case, the typical intersecting surfaces would be even closer to planar.
I first realized that this would work back in 2006, if I remember correctly. I had realized that one could get latitude from a lunar distance observation. Latitude from a lunar?! Say it ain't so. This works because the Moon's altitude is affected by parallax while a star's altitude is not. So the measured angle between a star and the Moon changes with altitude, and that means that the measured angle (a lunar distance) can serve as a proxy for altitude. It's low accuracy, but it gives the Moon or star's meridian altitude and the rest proceeds as normal: Lat = Z.D. + Dec. And all of this is done without measuring any altitudes. No horizon required.
The Moon is a bit far away for this process to be a valuable practical method, but once upon a time it was even considered an option for missile guidance. That idea didn't get very far! We can make the process more practical by bringing the Moon closer. We do this by launching artificial moons into low orbits. Getting a position fix by watching the position of an artificial satellite as it flies overhead is conceptually quite similar to determining cones of position by lunar distance angles. And it's even easier since we don't need a sextant.
Frank Reed