NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
Re: Constant altitude curves on an ellipsoid
From: Andrés Ruiz
Date: 2015 Aug 16, 10:12 +0200
Using latitude, longitude, GHA and declination, as coordinates of the two vectors, ones obtain the well knwnon equation for the altitude.
You can use the ellipsoidal coordinates.
Please share with us your reserach, it is very interesting.
From: Andrés Ruiz
Date: 2015 Aug 16, 10:12 +0200
Dear Robin,
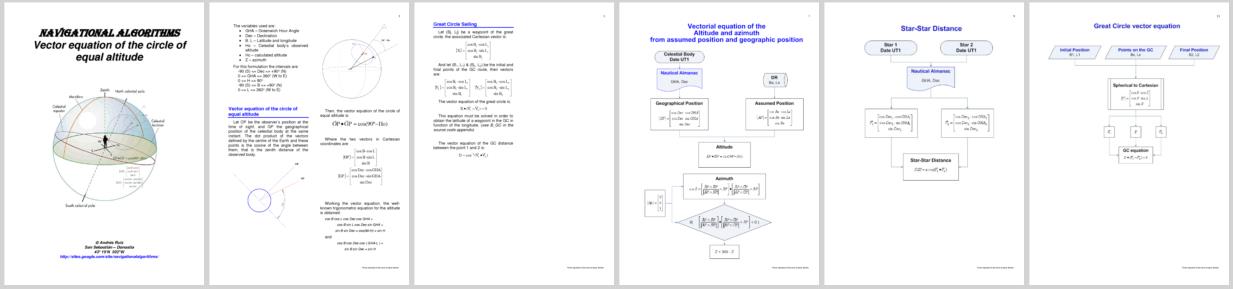
The equation of the circle of equal altitude in its vector form is universal, see the article "Vector equation of the Circle of Position" at Papers section in my Website.
OP · GP = cos( 90º-Ho )
OP is the observer’s position at the time of sight, and GP the geographical position of the celestial body at the same instant.
The dot product of the vectors defined by the centre of the Earth and these
points is the cosine of the angle between them, that is the zenith distance of the
observed body.
The equation of the circle of equal altitude in its vector form is universal, see the article "Vector equation of the Circle of Position" at Papers section in my Website.
OP · GP = cos( 90º-Ho )
OP is the observer’s position at the time of sight, and GP the geographical position of the celestial body at the same instant.
The dot product of the vectors defined by the centre of the Earth and these
points is the cosine of the angle between them, that is the zenith distance of the
observed body.
Regards,
--
Andrés Ruiz
Navigational Algorithms
http://sites.google.com/site/navigationalalgorithms/
...
I do not have the book “Geometry of Navigation” by Roy Williams, in amazon you must pay between $100 and $200.
Navigational Algorithms
http://sites.google.com/site/navigationalalgorithms/
...
I do not have the book “Geometry of Navigation” by Roy Williams, in amazon you must pay between $100 and $200.