NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
Re: The Great Circle Challenge
From: Peter Hakel
Date: 2014 Dec 26, 17:44 +0000
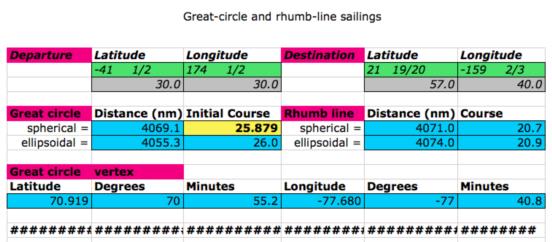
Initial course 026 - ignoring North Island being in the way. I don’t recall how long it took me to assemble that spreadsheet (it was a while ago) but it was relatively quick compared to some other ones. There indeed are some decisions (IF statements) coded in line 21 (no time to give more details right now). With that done once and for all, entering those four numbers took less than a minute.
Peter Hakel
From: David Pike <NoReply_DavidPike@fer3.com>
To: pmh099@yahoo.com
Sent: Friday, December 26, 2014 9:21 AM
Subject: [NavList] The Great Circle Challenge
From: Peter Hakel
Date: 2014 Dec 26, 17:44 +0000
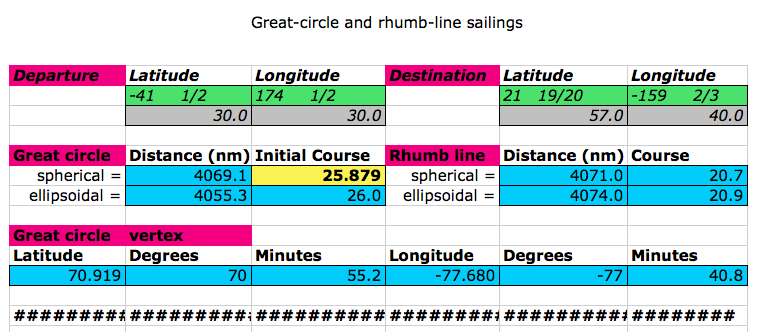
Initial course 026 - ignoring North Island being in the way. I don’t recall how long it took me to assemble that spreadsheet (it was a while ago) but it was relatively quick compared to some other ones. There indeed are some decisions (IF statements) coded in line 21 (no time to give more details right now). With that done once and for all, entering those four numbers took less than a minute.
Peter Hakel
From: David Pike <NoReply_DavidPike@fer3.com>
To: pmh099@yahoo.com
Sent: Friday, December 26, 2014 9:21 AM
Subject: [NavList] The Great Circle Challenge
The Great Circle Challenge
Clearly, from recent posts, we all have a favoured way of solving great circle sailings (which we’ll probably never use for real). Let’s put them to the test by trying something a bit more complicated like a southern to northern hemisphere crossing combined with a crossing of 180degrees E/W. How about emulating Captain Cook by travelling from Cook Strait (CS), New Zealand to Waimea Bay (WB), Kauai, Hawaii? Cook followed the pretty route, but we’ll go direct by great circle. The coordinates are CS 41d 30’S, 174d30’E to WB 21d57’N, 159d 40’W. Use your favourite method and report back on your answer, the time it took you, and any difficulties encountered.
I’ll stick with the diagram method, because at least I’ll know what I’m trying to prove, and I won’t have as many rules to remember and apply which might or might not work. Dave