NavList:

Ed Popko, you wrote:
"In reading past archive posts on Lunars, I came across an old thread discussing how LOPs can be derived from a lunar observations. The fact that a Lunar can be taken with no horizon makes this a useful method. How often do you have a clear sky but poor visibility of the horizon? It seems worth knowing about. And of course, if you can derive a LOP, you are likely to have other information from your DR or sights to derive a fix."

Yes, I put a lot of effort into explaining this, publicizing it, and coming up with methods so that modern navigators coul try it out. It talked about this methodology in a couple of presentations at Navigation Conferences at Mystic Seaport Museum back in 2008/2010. This was also a significant part of my talk at the NMM in Greenwich six years ago entitled "Lunars in the Space Age". This is, in fact, how lunars were used on the Apollo missions and later aboard the Skylab space station (never needed in either case, but they experimented with manual celestial navigation using sextants, and the angles they shot were most analogous to lunars).

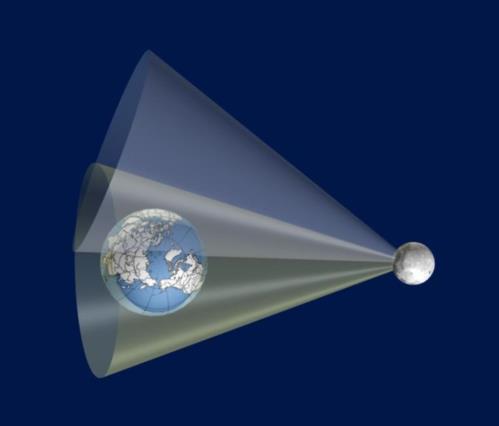
In three-dimensional space, any altitude sight that we shoot places us on a "cone of position". If I am at sea, and I measure the Sun's altitude above the horizon as 70°, we usually draw a circle of position centered on the sub-Sun point with a radius, 20°, equal to the zenith distance. But this circle assumes we are on or very near the surface of the Earth, usually an excellent approximation. But if we think a little deeper, that zenith distance could imply that we are on a surface extending up into space and (at least in theory) into the Earth's interior. The surface is a cone with its apex at the Earth's center and with a central angle equal to zenith distance. The intersection of that circle with the Earth's surface yields the usual circle of position that we all know so well. Note that as we climb higher into space on this "cone of position", the dip of the horizon becomes larger and larger, but the zenith distance is the quantity to focus on. If the zenith distance is 20° at the surface of the Earth, then it will be 20° at all points on a cone extending that circle up into space.

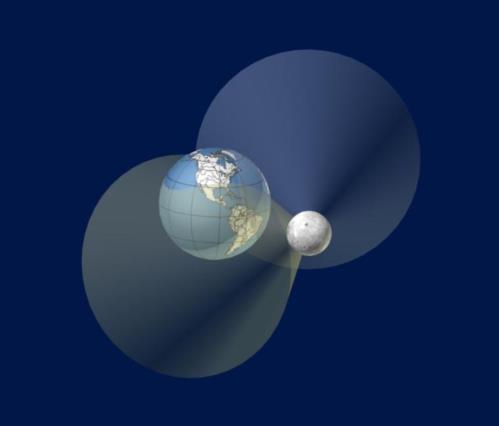
When we get quite far from the Earth, the idea of the "dip of the horizon" disappears. If you're high enough "up there", the Earth becomes a small disk in your sky. At that point, we would likely no longer think of dip of the horizon, but instead we would see the Earth as having its own semi-diameter, like the Sun or the Moon in our skies from here on the ground. Indeed for any object, whether its the Earth or Moon or some other body, there is a simple connection between SD and dip:
  SD + dip = 90°.
It's an odd thought and a surprising equation, until you realize that the Earth, even when we are standing on it, is a celestial body with an angular size. If my height of eye is 100 feet so that the dip is very nearly 10', then the SD of the Earth is 89°50'. It fills nearly half a hemisphere of the "sky" around us. Climb to 40,000 feet and the dip is now 2°20'. At that altitude, the Earth's SD is 87°40' and its at these altitudes that we can begin to detect the "roundness" of the Earth as an object. It still fills nearly a hemisphere in our field of view, but not as completely as when we are near sea level. Fly out into space and the earth might be, as an example, 30° in diameter or its SD is 15°. We could still, in principle, measure altitudes above the horizon and use a "dip" of 75° (SD+dip=90°).

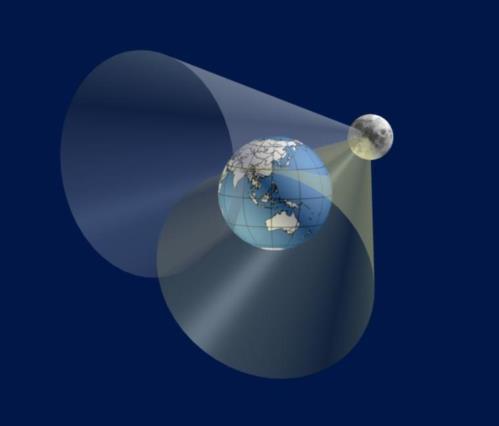
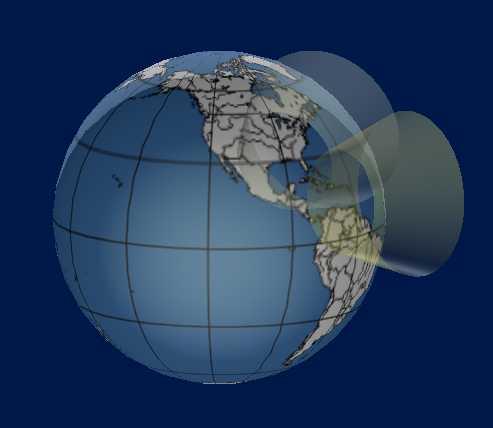
What does this mean for lunars? When you measure an angle between the Moon's limb and a star, you are, literally, measuring a celestial altitude, but it is the altitude above the Moon's horizon. That limb of the Moon is just the place where the "land meets the sky". In this case, it's where the "land" of the Moon meets the sky. Once we realize that lunar distance angles are completely analogous to altitudes above the terrestrial horizon (but with a huge dip), then we can analyze them using the same geometry that we apply to the three-dimensional analysis of sights on the Earth. Every lunar observation, at a known UT/GMT, places us on a circle of position on the lunar surface and which extends into space as a vast cone of position. In this case the apex of that cone of position is located at the Moon's center. From here on the Earth, these lunar cones of position are enormous things, and in the vicinity of the Earth, a small portion of one is barely different from a flat plane. Next time you shoot a lunar, you can visualize this plane by holding your hand out flat so that your fingers point to the Moon and your open palm points toward the diection to the other body in the lunar sight (the star or planet or the Sun). Your (flat) hand approsimates a plane. The lunar distance observation places you somewhere on that plane surface, and where the plane intersects the surface of the Earth, you have a locus of points where you as an observer might be situated. When you're holding your hand out in front of you, picture where it "cuts" the chart on th ground to visualize the lunar line of position (and to better understand its azimuth direction).

So how do we calculate this? Unfortunately a cone with its apex at the Moon's center extending into space until a portion of it intersects the Earth's surface is a rather messy geometric problem. Maybe someone could put together a "closed form" solution, but in an age of fast computation power, this would be rather pointless. Instead we can find all points on the locus of positions where the cone intersects the Earth's surface simply by repeatedly "clearing" the lunar and finding those places on the Earth where the solution is an exact match for our observation at the known UT/GMT.

It's worth emphasizing here how much this concept inverts the whole idea of lunars. Historically, of course, lunars were used to find GMT, and then from that the longitude. Lunars were one of the keys to longitude. They were all about longitude. But if we know the UT/GMT, as we almost always do today, then we can use lunars to fix our position on the Earth's surface, even our latitude, with no useful longitude information. Yes, you can get latitude by shooting a lunar. In fact, it's one of the easiest cases to understand...

Imagine the Moon in the sky maybe 60° high on the meridian due south of you. Meanwhile, also on the meridian below the Moon is the star Fomalhaut, and its altitude is 30°. You don't measure the bodies' altitudes (because of surface fog, perhaps) and instead you measure the angle between the Moon and Fomalhaut --a lunar distance observation. In the case as described here, the center-to-center distance turns out to be 30.000°, just for a nice round number. But now image travelling 25° to the north on that same meridian of longitude at the same instant of observation. Fomalhaut is now about 5° high and the Moon is about 35° high. We measure that lunar distance angle again, and it will be slightly different, perhaps 30.1234°. We correct for refraction, but there is still a difference. Any navigator who has dealt with Moon sights knows that this difference is due to the Moon's parallax in altitude. But from our new point of view, the parallax is not something to be corrected for, not some detail to be "undone". Rather the parallax is our friend. The lunar distance angle, that residual difference after correcting for refraction, is, in fact, telling us how high the Moon is. We are measuring the Moon's parallax and then inverting the usual relationship to get the Moon's altitude from it. And therefore, since the Moon is on the meridian by stipulation, the derived altitude gives us our latitude by the usual simple calculation: Lat =z.d. + Dec. Latitude from lunar distance! Note that this is the point of view of a ground-based navigator. If you can work in three dimensions and picture the cones of position, this idea of deriving altitude from parallax is un-necessary.

You contnued in your post:
"After clearing the initial lunar, I created a box around my Known Position based on the four plot sheet corners (rounded, plus/minus 30’ lat/lon) and reran the Lunar Calculator for each corner holding everything else constant (time, LD observed, body etc.). I marked the computed Lunar Error (LDe) at each corner. See the attached Small Area Plot Sheet. The dot “MAV” marks my known position. It’s Lunar Error, 0.1’, is also marked. Next, I found two points where the sign of the Lunar Error changes from plus to minus and marked them on latitude 41°30’ and 42°30’ lines. These points were trivial to find; they are marked and the resulting LOP is plotted..."

Yes, that's exactly how I have recommended working it up. You can imagine drawing a whole series of curves across the globe at any instant of UT. On one curve the measured lunar distance between some star and the Moon is 32°10.0'. Along a nearly parallel curve the lunar distance is 32°10.5' and along a curve on the other side of the first one, the lunar distance is 32°09.5'. Assuming the observed distance isn't badly erroneous, you only need to work up a small set of these curves in a "box" around your estimated position. You calculate the "error" at each of four corners around your estimated position and then you interpolate to determine the points where the error would be zero. Connect the zero points, and you have your lunar distance line of position.

Next, you should consider errors. An error of 0.1' shifts a lunar line of position like this by six nautical miles. That's because the center of the Moon is about 60x further away then the center of the Earth when we take these sights (about 4000 miles to the center of the Earth; about 238,000 miles to the center of the Moon). But it's not as simple as with terrestrial altitudes. Celestial navigators are spoiled rotten by beautifully simple geometry which allows us the simple rule that every minute of arc in altitude corresponds to exactly one nautical mile on the ground (it's not really exact, but it's damn close). This rule does not apply to lines of position from lunar distances. Instead picture that plane intersecting the Earth's surface. Again, you can do this by holding your hand out flat, fingers pointed at the Moon's center, normal vector to your palm pointed at the star. The pam of your hand corresponds to the nearly planar surface of position determined by the lunar observation. If the observed angle shifts by 0.1', that surface shifts up or down (your palm moves towards or away from the star) by six nautical miles. The resulting error in the lunar distance line of position will be determined by the intersection of that plane with the ground. If the Moon is very high in the sky, then it's more or less one-to-one: six miles shift in the plane surface yields six miles on the ground. Or if the Moon is low in the sky, and other body is at a similar altitude to the left or right of the Moon, then plane surface shift also yields a nearly one-to-one match on the ground. But if the Moon is low in the sky, and the other body is above it on more or less the same azimuth, then when your hand shifts up or down by six miles, the intersection point moves by many miles (approximately 6/sin(alt)).

You can get a fix from a pair of lunar distance measurements at the same UT under many circumstances, or if the geometry is unfavorable, from a pair of lunar distances separated by a few hours. Imagine you're in the tropics, and the Moon is at high altitude. You measure a lunar angle from one star that is directly below it in the south and then another lunar angle from a star in the east or west. That would yield a pair of lunar distance lines of position crossing at a wide angle, and you would have a fix (low accuracy, +/-6 miles at best, but still a fix). Next imagine you're in high latitude, and the Moon is very low in the sky. you measure a lunar angle for a star to the left or right of the Moon. This produces a lunar line of position with the usual +/-6 n.m. accuracy. But if you attempt a lunar distance measurement for a star directly above the Moon, the results on the ground would be quite poor. Instead, wait a few hours. After the Moon has moved 30° or more in azimuth, shoot the same star-moon distance as before. You'll get a new lunar line of position crossing the first. You get a running fix from that.

Finally, note that this methodology of deriving a position fix from the Moon is geometrically identical to the problem of getting a fix by observing artificial satellites. But the practical differences are huge. All easily-observed artificial satellites are zipping across the sky at a rate far too rapid for sextant obsrvations. On the other hand, bright  satellites are never so bright that they overwhelm the light of nearby stars (as the Moon does) so we can easily determine the exact location of an artificial satellite among the background stars without a sextant. And also, again since artificial satellites are moving so fast, it's better to treat time as somewhat uncertain which generates yet a different sort of line of position on the ground. But this is a story for another time...

Frank Reed

File:

File:

File:

File:

File:

File: