NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
From: Hanno Ix
Date: 2015 Feb 22, 20:34 -0800
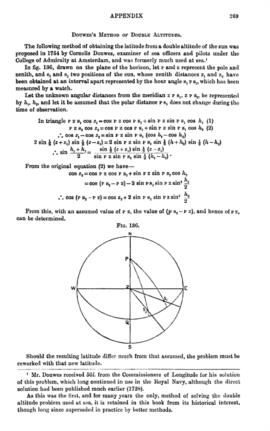
A treatise on navigation and nautical astronomy By William Robert Martin
and attach copies of the pertinent pages. Is this the issue you are referring to when you speak of Douwes Problem?
If not would you be so kind to describe the problem and its solution?
Regards
H
Hello to all
We all know that the Marcq Saint Hilaire's LOP's method requires some approximate knowledge of one's position. Such position should be carefully "maintained" and for this reason devoting all one's care to DR computations has remained the backbone of our classical "on account" navigation. We have been taught that such DR position is a requirement to fuel up the computations pump: whether a Classical Sight Reduction Table, or abriged methods which have become quite popular here lately or even - horresco referens ! - processing LOP's through a calculator of some kind.
But WHAT IF if you do not know at all your DR position ???
With at least 2 observations adequately spaced in Azimuth, can you still process your position without some approximate preliminary knowledge of your position ? ?? ???
Apparently one similar problem was raised by Dutch Astronomer Cornelius Douwes in the first part of the 18th century. Obviously he was not aware of the celebrated Marcq Saint Hilaire's method which was to be discovered surprisingly VERY LATE (1875 only) given the simplicity of its concept. Nonetheless Cornelius Douwes is credited to have been the first one trying to sort out one's position at sea without preliminary knowledge of any DR position. Douwes even published some tables to that aim. For this reason the french naval documents usually list this problem as the "Douwes's Problem".
For many years, no solution to this problem has been published.
However as most of us would now know, the so-called "Douwes's problem" has been adequately solved through various manners, whether through "direct" methods or through "iterative" methods.
The following internet reference (http://ecole.nav.traditions.free.fr/officiers_bodenez_georges.htm) tells us that French Navigation Professor late M. Georges Bodenez was the first one to publish in France one such method. His method was published in one issue of the "Navigation" Magazine in 1976 by Institut Français de Navigation.
The exact and "direct" mathematical solution for 3 simultaneous LOP's on a Sphere has been published much later in one other "Navigation" issue probably some 20 (?) years ago. Incidentally, while a number of "Sailors" spent some efforts on this subject, this published solution in "Navigation" is to be credited to a French Air Force Colonel.
Among current NavList Members I am aware of at least 3 different Members who independently (re)discovered at least one such method to process at least 2 LOP's without any preliminary knowledge of some approximate position. All of them routinely use it and/or have published their (re)discoveries.
What I am interested here is not actually the different methods currently in use, whether the "direct" or the "iterative" ones. I am rather interested in the History of such discoveries and/or (re)discoveries.
What can be said is - like the Marcq Saint Hilaire's method - the currently published solutions to the Douwes's problem are no immensely difficult, especially as regards the iterative methods. For this reason, it is very surprising that the first such method officially recorded was published so late, i.e. less not even 40 years ago.
In other words, I am just curious to know whether some adequate solution(s) to the Douwes problem has been published elsewhere before the one published in France by Professor Georges Bodenez in 1976. Not to tarnish M. George Bodenez's Great Memory and Honor, but it so happened and will happen more than once in the history of Science that discoveries have been forgotten - probably because of low practical interest - and have been subsequently and independently re-discovered when the solution to some problems was needed. As well known examples, very many Math problems solved by Carl Friedrich Gauss were independently rediscovered and solved well after he left our World.
When, by whom and where ?
To you Paul Bedel, as you seem to be quite knowledgeable in the history of Celestial Navigation, would you be able to shed some light here ?
Thanks in advance to who-ever would be able to bring additional information on this topic.
Best Friendly Navigational Regards to all,
Kermit
Antoine M. "Kermit" Couëtte