NavList:

"I complete the Frank Reed's topic of May 2004 after a long research about the formula:
P = HP*{y + 0.000145*HP*cot D*[(cos h_moon)² – y²]} 
that appeared in an article on the lunar distance but that I did not understand. I didn't know how to use it either.
I finally came across George Huxtable's text:
https://www.siranah.de/sources/About_Lunars_by_George_Huxtable_2002.pdf
which led me to John Letcher's and I had the explanation I was looking for."

It sounds like you took a rather long and circuitous path to get to this. You should have said 'hello' and started a conversation here about this much earlier. I think you could have saved a lot of time and learned more, too! The archives of the NavList community are, unfortunately, seductive that way. 

You have here posted some rather long mathematical analysis connecting Letcher's slick, efficient formula,
   P = HP*{y + 0.000145*HP*cot D*[(cos h_moon)² – y²]} ,
with the convoluted, idiosyncratic math found in Chauvenet. You have certainly successfully shown that these two very different mathematical treatments are "consistent". That's no surprise, I hope. Right? They have to be consistent --but that doesn't imply a genetic connection. Your analysis actually may be very useful to those navigation enthusiasts, including some NavList members, who are intrigued by Chauvenet's methodology since it will help tie that peculiar, late 19th century method to the earlier tools (which had already solved the problem long before Chauvenet started worrying about it).

I have to say that I don't agree at all with your speculation that Letcher might have done the same thing --that he might have derived the formula he published in his book by such a long and painful reverse-engineering of Chauvenet. There's simply no evidence for that. Nothing at all. And besides, anyone with the kind of math background that Letcher had (a physicist by training) could have derived this equation from scratch. It requires little more than very basic spherical trigonometry knowledge and skill with "small angle approximations". It's a series expansion (and that's "bread and butter" stuff in physics and astrophysics). Letcher may have had some hints from an old edition of a navigation manual from the period from c.1770-1850 (when lunars were actively used at sea), or he may have simply worked it out from first principles after he learned about the general concept in secondary sources. There was a long "school" of series methods developed and enhanced by Lyons, Bowditch, Norie, Thomson and many less successful creators. Letcher could have had access to any old book with these methods and tables available for his analysis.

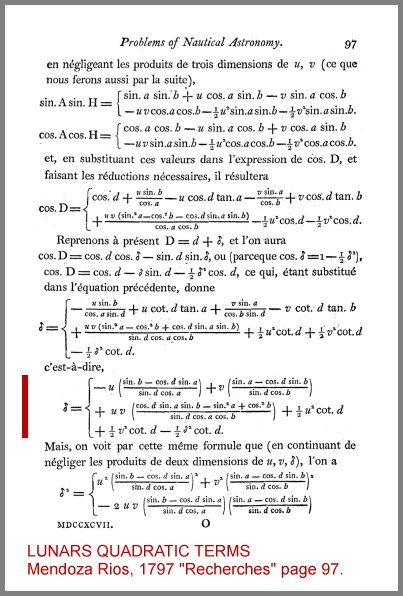
That "quadratic term" in the analysis of lunars dates back to the 18th century, at least in print. One of the most famous sources from the era was a long and detailed article from 1797 on mathematical topics in nautical astronomy by Josef de Mendoza y Rios (a Spanish mathematician who lived in London, spoke several languages, and wrote in Latin and French). This was published in the Transactions of the Royal Society, easily the most prestigious and influential scientific publication at that date. I'm attaching (another) pdf copy of this article here. The section on the series expansion is near the middle of the article. The math is old-fashioned and confusing at first, but that's part of the fun, right?

Frank Reed

File: