NavList:

Hello Antoine, quoting from your message:

———————
As an example, with the following numbers rounded to integer values let's us start from :
- Tony's Position (i.e. from position N60° E030° , Hello Tony ! ) , and :
- Dave's Height ( 55° i.e. a sub-astral distance D = 2100 NM ),  and :
- an Azimuth Az = 030°,
we get a subastral point at N73°17.4' E124°06.6' hence with LHA = 94°06.6'
———————

In the following I consider the GP fixed at this first location that you gave, and look for the position(s) from which this celestial body can be seen at the given altitude (55°) and azimuth (030°). To that end, I modified my “one-body fix” spreadsheet to process both allowed LHA values. I get these two solutions:

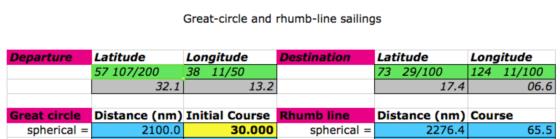
1) 57° 32.1’ N, 38° 13.2’ E
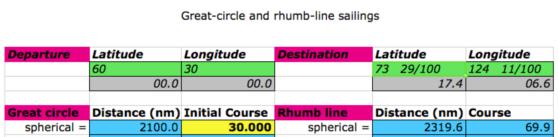
2) 60° N, 30° E

They both give the correct azimuth = 30, as well as the correct computed altitude of 55° (i.e., distance of 2100 NM, see attached), so there is no way to choose one solution over the other without additional information. This looks like the ambiguity mentioned in the Wikipedia link provided by Dave Walden, because here the known (azimuth) angle is attached to the longer side of the triangle (ZD=35° > coDec~17°). BTW, as expected, my original spreadsheet gives solution 1); the “Tony” solution 2) comes from the added, larger LHA path.

Peter Hakel
https://www.navigation-spreadsheets.com/navigation_triangles.html#sailings

File:

File: