NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
From: Frank Reed
Date: 2018 Apr 4, 19:06 -0700
You have no almanac or app handy, and you want to estimate the Sun's altitude at noon. You're good with noon sun sights for latitude, so you know the basic equation:
Lat = Z.D. + Dec.
We need to turn that into an equation for altitude. It's not hard to see:
Alt = (90° - Lat) + Dec.
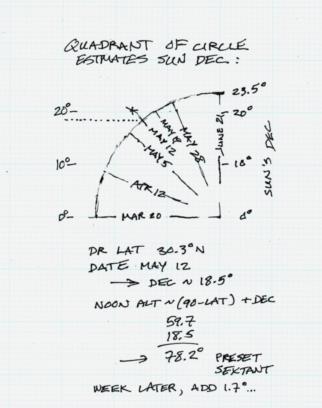
So we're all set if we can get an approximate value for the Sun's declination. To do that, draw a quadrant of a circle. The "y-axis" up the side of the quadrant represents dec from 0 to 23.5°. Label the sides with the dates of the equinox and solstice that wrap the date you're interested in. Then the angle at the center of the quadrant (corner of it really) represents the dates in between. Split the quadrant up into at least four sections, and you can easily estimate the dec for your chosen date. There's an example in the attached diagram. Note that this was sketched on graph paper (a little hard to see in the scan), which is key to reading off the numbers. I find that I can get the correct dec and from that the correct noon altitude within a half degree using this method. That's plenty good enough for presetting a sextant or for other planning purposes.
You can use the same quadrant diagram to estimate the rate of change of dec. Label the bottom side from 24' at the end of the March 21 line to 0' at the corner of the quadrant. Find the date you want and you can quickly read off the daily change in minutes of arc from that side of the diagram.
Frank Reed