NavList:

Traditionally lunar distance tables in the almanacs were computed and tabulated by GMT (UT today). For example on March 31, ten days from now, at 15:00 GMT, the Sun-Moon lunar distance will be 80.3830°, and at 16:00 GMT the distance will be 80.8741°. If I observe a lunar distance, clean it up to remove the effects of refraction and parallax (called "clearing" the distance) and find that my distance is 80.63°, then I can deduce that the GMT is just about 15:30 since my observed distance is about halfway between the two tabulated values. 

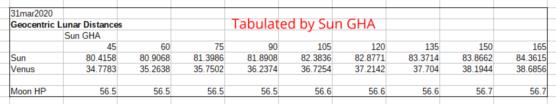
Suppose instead of tabulating by GMT, we create an almanac that lists the lunar distances with the Sun's GHA as the independent variable (example for part of March 31 attached). What would be the advantage? The table would show the predicted lunar distance for specific values of GHA. Observing a lunar would directly yield the Sun's longitude -- its GHA. It's as if the lunar is directly providing our normal almanac data. We immediately deduce the Sun's GHA from the observed lunar distance. Then a quick time sight yields the HA or "hour angle" of the Sun which is exactly the same as the difference in longitude between the observer and the Sun. Then:
   Longitude = GHA + HA
(GHA - HA if the Sun or other body is west of you, altitude falling). Quick and easy.

Let's do one. I observe the lunar distance with a sextant, clear it and get 80.63° (same as above). I look in the tables and find that the distance was 80.4158° when the Sun's GHA was 45°, and the lunar distance was 80.9068° when the Sun's GHA was 60°. The observed value is about a third of the way from the lower to the higher so just by rough estimation, we know that the Sun's GHA was about 50° when I measured the lunar (a third of the way from 45° to 60°). When we do the interpolation math, we find that the correct GHA is 51.54°, not too far from the first guess. This is the Sun's GHA right at the time of that lunar shot. If we shoot the Sun's altitude at the same time, we can immediately process the sight without looking up the GHA in an almanac. The lunar has already provided this bit of almanac data to us.

You don't even have to think about GMT when the lunar distances are listed by GHA. Of course, you could do the same by getting the GMT from the standard tables and then calculating the GHA from that GMT, but that adds extra work both in computing and conceptually. Why not just tabulate the lunar distances with GHA as the independent variable?

So we should do that, right? Convenient, right?

Too late... They already did it this way for two-thirds of a century, in the early decades of lunars. The early nautical almanacs tabulated the lunar distances by GAT, Greenwich Apparent Time, which is "sundial time" at Greenwich. In fact, GAT is identical to GHA but expressed in hours, minutes, and seconds instead of degrees. If the GAT is exactly 2:00pm, then the GHA of the Sun is exactly 30°. And I do mean exactly. When you look at lunar distance tables in the early era, from 1767 to 1833 (in the British Nautical Almanac and Astronomical Ephemeris), the distances are actually tabulated by GAT, and this is the same angle as our modern GHA, just expressed in different terms.

Frank Reed
Clockwork Mapping / ReedNavigation.com
Conanicut  Island USA

File: