NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
Re: The backstaff. was: Re: The Shovell disaster
From: Nicolàs de Hilster
Date: 2007 Nov 07, 10:24 +0100
From: Nicolàs de Hilster
Date: 2007 Nov 07, 10:24 +0100
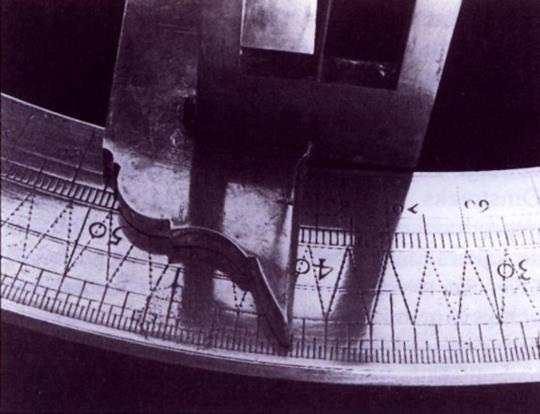
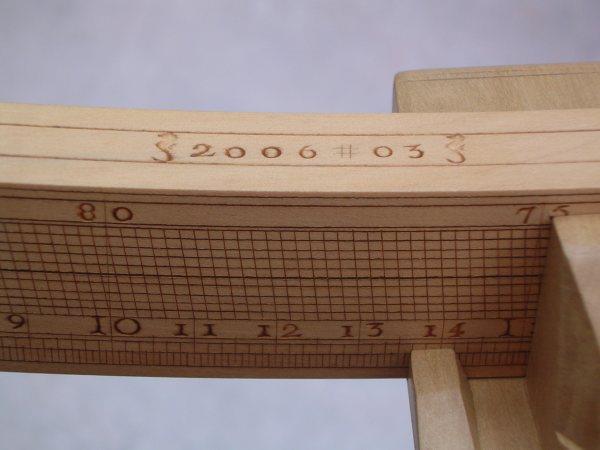
To keep it simple I will answer NavList 3755 & 3810 (Michael Daly) and 3812 (George Huxtable) in this single post. In Navlist 3755 Michael wrote: > Circular arcs require circular > transversals to be accurate. However, it was difficult to figure out > how to construct them (one technique was determined by Philippe de La > Hire and used by Nicolas Bion) and very tedious to construct (I have > created a diagram of linear transversals on Wikipedia but have yet to > tackle the effort of making a circular transversal diagram - yet I have > the advantage of a CAD program, not a sheet of brass with dividers and > compasses). So far I did not yet answer to this correctly, so I will give it a try: There are two ways to construct those diagonals: 1) Using a tilted cutting plate (I think that is how they were constructed) 2) Using a very long beam compass And now in detail: 1) Using a tilted cutting plate (I think that is how they were constructed) When I make the diagonal scales I use a beam with a cutting plate along which I cut the lines of the scales. The position of the beam is controlled by a series of holes along an arc outside the actual scale (the instrument is fixated on a board on which that arc is). I start out with the perpendicular line as those are the easiest. Then the concentric lines are drawn and small marks are made on the first and last concentric circle to indicate the positions of the 10 arc minute intervals. Not all 10 arc minute intervals are marked, just a few of them. The divisions of the whole 5 degree marks are drawn as well. Now the cutting plate is tilted using two adjustment screws until it fits the small marks that I made before. With the cutting plate in the proper direction I can mark of the diagonal scale in the smae way as I did the perpendicular scale. 2) Using a very long beam compass John Bird left us some instruments on which can be seen that the degree marks were cut using a beam compass, a method that could be applied on making diagonal scales as well (see attached picture, sorry for the quality, it comes from ION Vol. 34, No.2, /Moskowitz: World's First Sextants/). Clearly can be seen that the divisions are curved and that the centre of the beam was on the left. In his book '/Taking The Stars/' Peter Ifland says "...a more accurate construction required that the diagonals be arcs of a circle passing through the point where the diagonal crossed the base line of the scale and the center of the instrument." (p. 52). This method too is just an approximation of the problem as the diagonals are no arcs but clothoids. On the W. Garner instrument the approximation is however quite good. When drawing an arc through the actual required crossings on the scale it misses the centre of the instrument by only 0.4 millimetres. That is when creating 10 arc minute diagonals. For 20 arc minute diagonals it misses the centre by 3.2 millimetres. The problem in constructing the beam compass for this job is calculating the length of it. So far I can only say that for 10 arc minute diagonals the beam compass should be 4.40 times the largest radius of the concentric circles (which equals 2.612 meters). For 20 arc minute diagonals this should be a factor 2.28 (1.355 metres). Both values come from the reconstruction in AutoCad. Beam compasses this size have been used in the past for mural quadrants by John Bird and had as disadvantage that they grew and shrunk with temperature and humidity (these problems have been described by him in a book, I believe it was 'The Method of Dividing Astronomical Instruments', dated 1767). How to calculate the beam compass in a simple manner is another question. The calculation depends on the diameter of the instrument, the intervals on the diagonal scale and the width of the diagonal scale (so the distance between the inner and outer concentric circle). Using trial on error is probably a better option. The advantage of using a beam compass is evident: one gets the properly shaped diagonals (when using it from the right side, right meaning not left). In NavList 3810 Michael wrote: > I guess the AutoCAD construction shows that for those instrument sizes, > the difference between linear and circular transversals is irrelevant. > > Yes, that is correct. I did the same exercise for a 20 minutes diagonal scale which as expected doubled the error: 20 arc minute diagonals decimal radius [mm] error [mm] error [secs] 0 579.0 0.0000 0.0 1 580.5 0.0081 2.9 2 582.0 0.0143 5.1 3 583.5 0.0188 6.6 4 585.0 0.0215 7.6 5 586.5 0.0224 7.9 6 588.0 0.0215 7.5 7 589.5 0.0188 6.6 8 591.0 0.0143 5.0 9 592.5 0.0081 2.8 10 594.0 0.0000 0.0 Even here the largest error is only 8 arc seconds, nothing to worry about. Then in NavList 3812 George Asked: > Why can't the diagonals of such a diagonal scale be drawn simply as exactly > radial lines, and then the edge, against which they are read, be angled > correspondingly instead? Am I missing something? > That sounds like the perfect simple solution: only one diagonal to be made! I can think of three reasons why it was not done that way: 1) Due to historical development 2) Because a straight edge was needed anyway 3) Because of alignment problems Let me try to explain it in detail: 1) It was not done due to historical development The first diagonal scales were made of zigzag lines around the scale. Attached you will find a picture of such a diagonal scale on a Hollandse Cirkel (a Dutch land survey instrument). This design is older than the one we know from the Davis Quadrants and as you can see it leaves no room for the option George suggests. Then that new design came up and the basic principle stayed the same: the diagonals on the scale and the reading edge straight. 2) It was not done because a straight edge was needed anyway Attached you will also find a detailed picture one of the Davis Quadrants I made. The diagonals are clearly visible and on the right is the inner side of the sight vane with the straight edge that was needed to take the readings. As you can see along the lower edge of the scale there is a 'normal' perpendicular scale divided into 5 arc minutes intervals. For that part one would need a perpendicular edge to read it (like there is on this sight vane). So in order to combine the two one had to make a kink in the edge so that the lower part would be perpendicular and the upper part diagonal. The diagonal then would have to point to the right to make it possible to read the scale, but that would also add to the confusion as the diagonal would read ahead of the scale. One could make it the other way around as well (so mirroring the whole vane design) but then the diagonal would seem to read behind of the actual value. 3) It was not done because of alignment problems Lets suggest we would have made the scale as in 2). Any wear of the inner side of the vane, or outside of the scale would let the diagonal of the vane move inwards towards the centre of the instrument. As a result of this the readings will not be correct any more. Another thing is that when constructing the Davis Quadrant the arc is sawn from a piece of wood. The shape is drawn onto it using a beam compass, but there is no 'centre of the instrument' as yet, only the diameter is known. Then the whole frame is constructed and the instrument gets its centre, which is a small hole drilled into the main beam on the observer side of the horizon vane (the surface of the horizon vane is the centre of that hole). Small errors made during the construction phase of the frame will result into a misalignment of the outside of the arc and the centre of the instrument. As long as this error is small it will not affect the readings of the diagonal scale (when made as we know it). The concentric circles will not be completely parallel to the outside of the arc, but that can be compensated by tilting the vane until the edge aligns with the scale (something that has to be done anyway, regardless the quality of the concentricity). What does change is the distance between the concentric circles and the outside of the scale. So if constructed the way George proposed the diagonal would be in the proper position at one end of the scale, but not when on the other end of the scale and therefore introducing errors. At the end this post has become somewhat larger than expected.... Nicolàs --~--~---------~--~----~------------~-------~--~----~ To post to this group, send email to NavList@fer3.com To , send email to NavList-@fer3.com -~----------~----~----~----~------~----~------~--~---