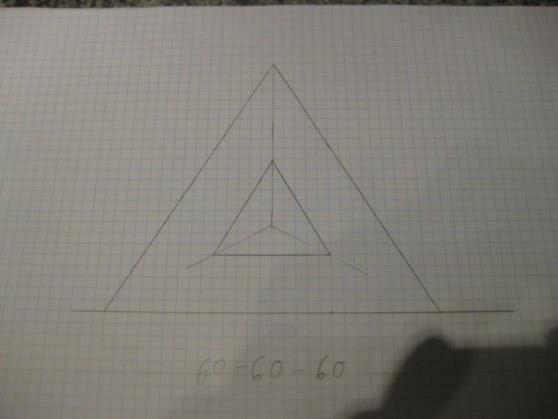NavList:

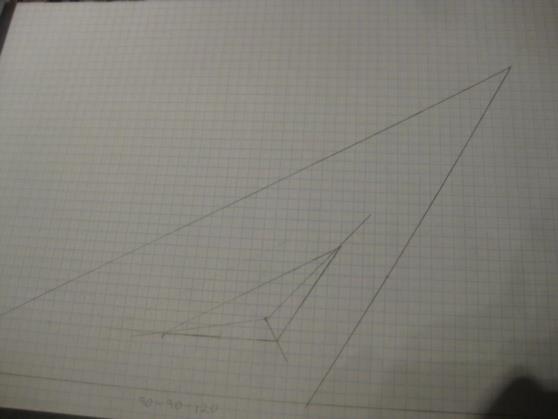
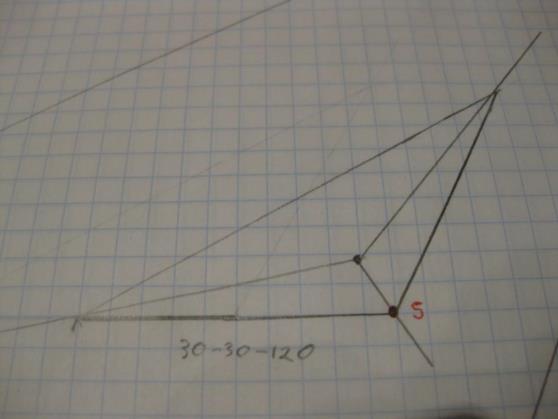
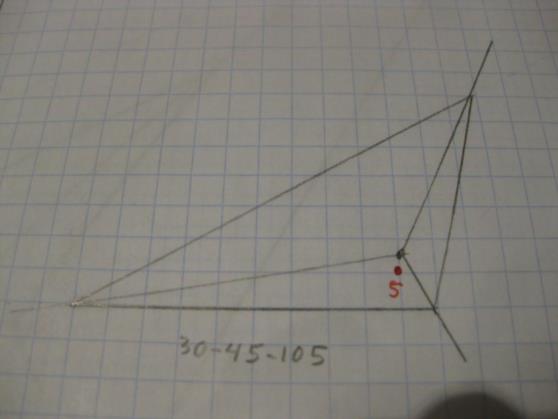
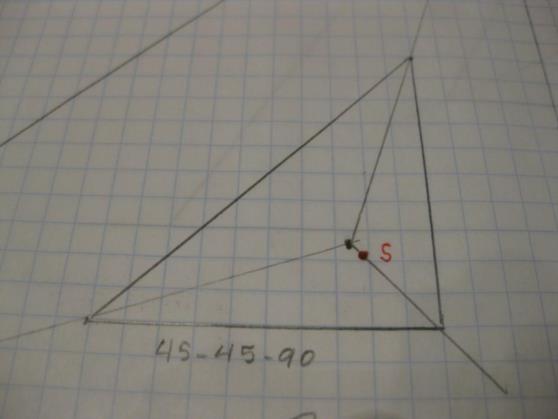
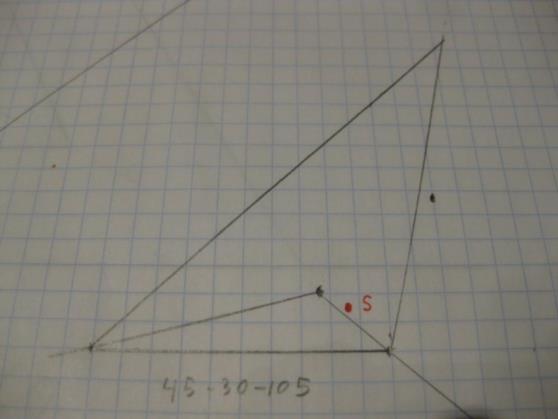
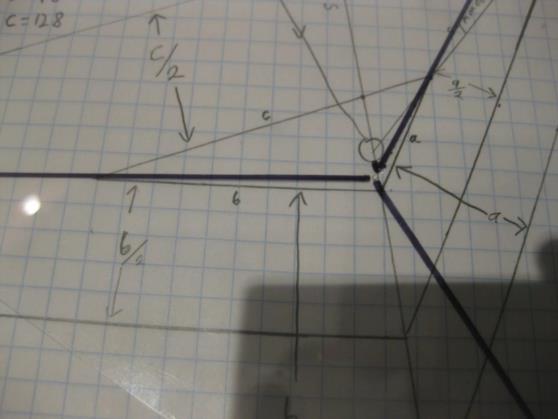
To make certain that this dead horse has been thoroughly beaten, I decided to draw several diagrams so that we can compare two methods of approximating the symmedian point. (This also assumes that Herbert is correct, that the symmedian point is the most desirable point to choose to represent the fix.) The two methods illustrated are the steiner point and the incenter. The steiner point is found with a template with three lines spaced 120 degrees apart. The incenter is found by drawing the intersection of the bisectors of the vertexes. (Or easier, by bisecting the azimuths.) I didn't illustrate the centroid method (found by drawing in the medians) since it is farther away from the symmedian than the incenter, even though it is easier to construct than the incenter.

See:

https://www.NavList.net/m2.aspx?i=114986&y=201012

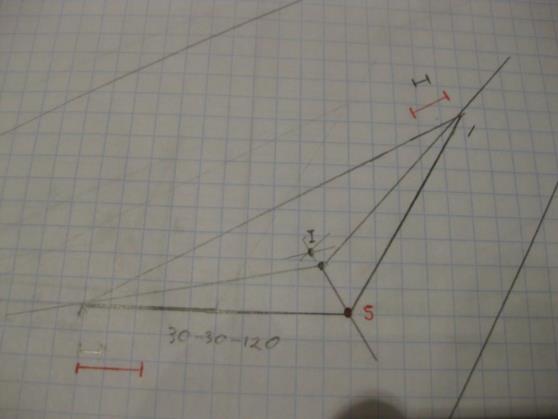
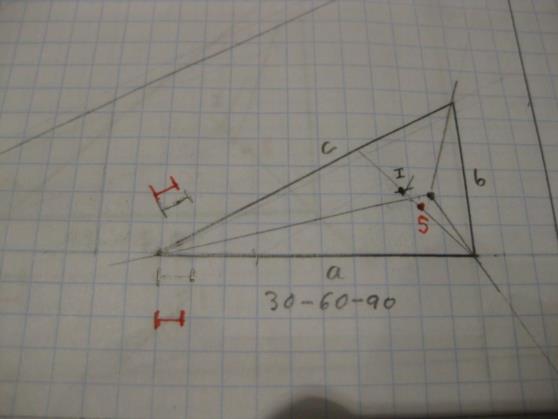
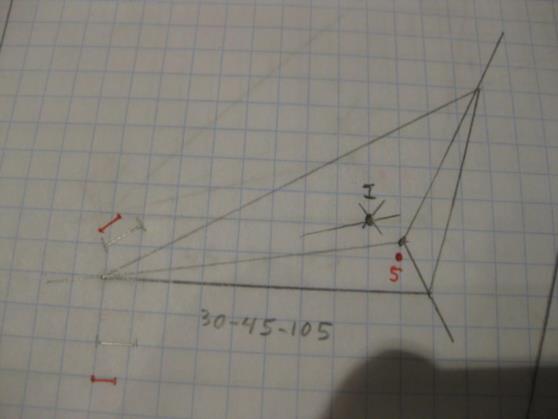
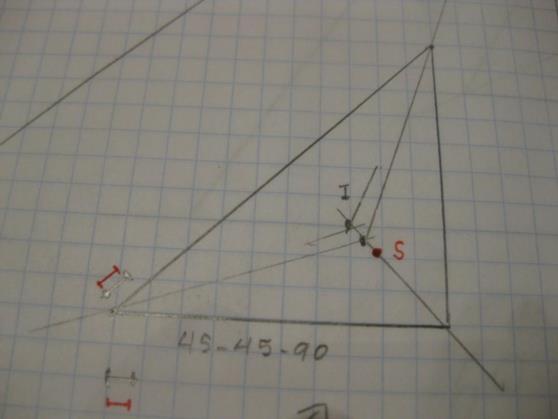
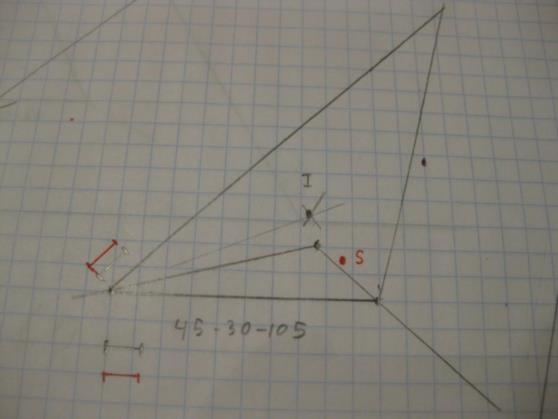
I limited the triangles to those normally used for a three body fix, all the cuts are 30 degrees or more. The first series shows six cocked hats from 30-30-120 to 60-60-60. These show the construction of the symmedian point using Herbert's method. The symmedian points are the unlabeled points at the intersection of the symmedian lines.

The second series shows the steiner points marked in red and labeled with an "S." These were found with a template.

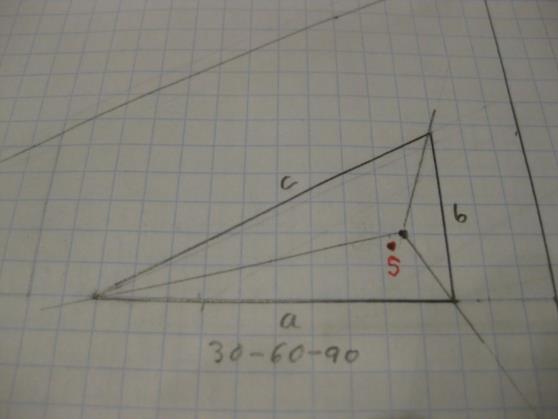
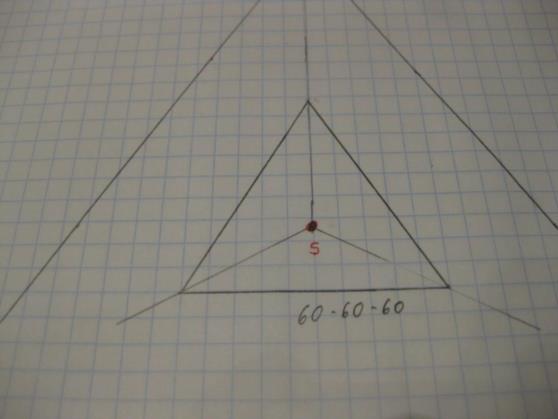
The third series shows the incenter marked with a black dot and labeled with an "I." You can compare the accuracy of the two points for approximating the symmedian point. To make this comparison easier, I drew the lengths of the space between the symmedian points and the steiner and the incenter points in bars next to both the longest side and the shortest side of each cocked hat, the incenter in black and the steiner in red.

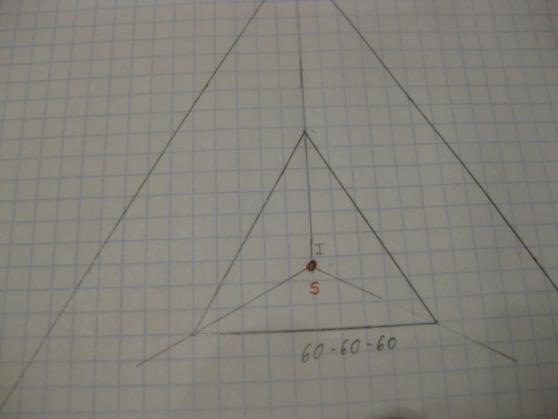
For the unique case of the 60-60-60 triangle all the points are the same, symmedian, steiner, incenter and centroid. For all the sample triangles except one, the 30-30-120, the steiner point is a better approximation of the symmedian than the incenter and is a lot easier to construct by use of a template.

So, take your pick.

(I have also attached an illustration of how the steiner point is found with a template.)

gl

----------------------------------------------------------------
NavList message boards and member settings: www.navlist.net/NavList
Members may optionally receive posts by email.
To cancel email delivery, send a message to NoMail[at]navlist.net
----------------------------------------------------------------

File:

File:

File:

File:

File:

File:

File:

File:

File:

File:

File:

File:

File:

File:

File: