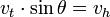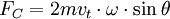NavList:

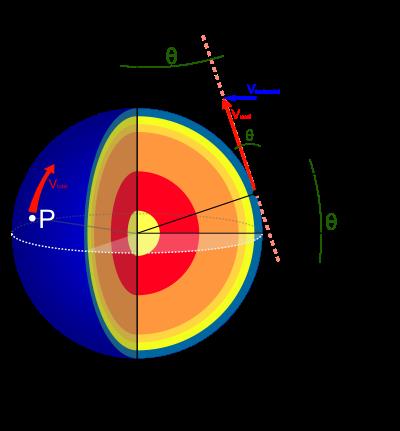
I don't claim to be a physicist but what I know about coriolis is that it creates an apparent or fictitious force on an object moving across a rotating reference frame, in our case the earth. This force is proportional to the speed over the ground and it is also proportional to the sine of the latitude. So, a gyrocompass in a ship tied to the dock located at the equator should not experience any coriolis force on two counts. First, it is not moving across the surface, and second, the sine of zero latitude is zero and zero times anything is also zero. Yet a gyro compass will quickly seek north when tied to a dock located at the equator so it can't be ol man coriolis doing the deed.

The common formula for calculating the coriolis correction to bubble sextant observations is Z = 2.62 times the ground speed (in hundreds of knots) times the sine of the latitude.The 2.62 is a just a constant to connect ground speed in hundreds of knots to the correction in nautical miles (or minutes of deflection of the vertical if you want to think of it that way. )  Using a bubble sextant on the ground requires no correction for coriolis because the ground speed of a person standing in his backyard is zero and zero times anything is still zero. Nor could coriolis be the explanation for he north seeking ability of Douglas' theodolite even though he is located 51º north of the equator since his theodolite also is not moving.

The common illustration for coriolis is someone rolling a ball across a turning merry-go-round but if he does not roll the ball but just keeps it in his hand then there is no coriolis because the ball is not moving across the turning reference frame.

See the attached links.

gl

	Visualisation of the coriolis effect. In the inertial frame of reference (upper part of the picture), the black object moves in a straight line. However, the observer (red dot) who is standing in the rotating frame of reference (lower part of the picture) sees the object as following a curved path.
	How the coriolis effects work The red line is an object on earth travelling from point P at the speed Vt (V_total). For the coriolis effect only the horizontal speed Vh (V_horizontal) is of importance. To get Vh from Vt one can say: The coriolis force is:

http://scienceworld.wolfram.com/physics/CoriolisAcceleration.html

http://scienceworld.wolfram.com/physics/CoriolisForce.html





frankreed@HistoricalAtlas.com wrote:

As Douglas Denny stated originally (later withdrawn), the Coriolis acceleration is most definitely responsible for the north-seeking capability of a gyro-compass (IF you work in coordinates rotating with the Earth --the natural coordinates for these problems). Just make sure you use the full three-dimensional vector cross-product when thinking about the strength of the Coriolis acceleration. 

The Coriolis acceleration is frame-dependent --it depends on the choice of a rotating frame of reference. It's intriguing to note that there is also an extremely tiny physical, non-frame-dependent, version of the Coriolis acceleration which is created by spinning masses. This is known as "frame-dragging" or "gravito-magnetism" and was measured (barely due to various problems with the ultra-sensitive gyroscopes) by a spacecraft known as "Gravity Probe B": http://einstein.stanford.edu/highlights/status1.html.

There is a deep, non-accidental analogy between the Coriolis force and the common magnetic force. And just as a spinning current loop experiences a torque which causes it to precess in a constant magnetic field, a spinning "mass loop" (a.k.a. a gyroscope) experiences a torque which causes it to precess in a rotating frame of reference.

Finally, for Gary, there is nothing "fictional" about the so-called "fictitious forces" in rotating frames of reference. They are every bit as real as the common acceleration of gravity, or, if you like, they are every bit as un-real. The complicated explanations for various phenomena which you have learned that avoid using the expression Coriolis are un-necessary, though they can serve as useful reminders that physics can be done in different frames of reference and the results have to be the same no matter what.

-FER





--~--~---------~--~----~------------~-------~--~----~
NavList message boards: www.fer3.com/arc
Or post by email to: NavList@fer3.com
To , email NavList-@fer3.com
-~----------~----~----~----~------~----~------~--~---