NavList:

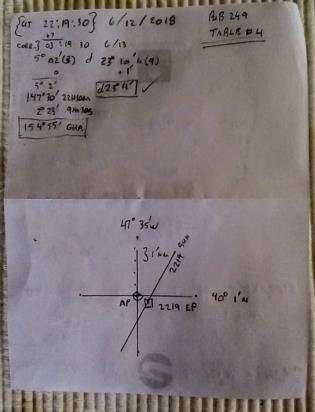
Attached working notes/plot for paper sextant sight example. Sight reduction by wallet HavDoniol natural haversine table, Ix azimuth diagram, and Pub 249 table 4 long term Sun almanac.

Greg Rudzinski 

From: Frank Reed
Date: 2018 May 6, 10:59 -0700

I wrote previously:

It's June 12, 2018. You are becalmed in the North Atlantic. You don't have a sextant or a Nautical Almanac, but you have a good wristwatch set to UT (GMT) and you have a calculator and some other basic items aboard (a rubber ball might come in handy here). So you make a paper sextant out of an index card (as in the image). You get the Sun's dec from the circle diagram I described a while back. You can estimate the Sun's GHA by knowing that the equation of time is near zero on this date (you knew that, right?). As the Sun is heading toward sunset, you measure its altitude above the sea horizon as 2° 30' +/-10'. The time on your watch is 22:19:30 GMT. Your height of eye is 10 feet (or 3 meters). And to make life a little easier, let's suppose that you have memorized an approximate refraction table and it's about 15' at this low altitude. Can you get a line of position from that sight? Suppose your latitude is 40.1° N, what is your longitude? Suppose your latitude is 40.2° N, what then is your longitude?

I've left enough time for folks to ponder this. Time to start working it up. You measured the Sun'a altitude (Lower Limb, of course!) as 2°30' with your paper sextant. Dip correction is about -3', refraction is about -15', SD is 16' as usual, so the corrected altitude (or "Ho") is 2°28' (and given the likely uncertainty in the altitude measured with a paper sextant, you could round this). Next we need the Sun's Dec and GHA. Dec we can get from the circle diagram (see earlier post). From that I get a Dec of about 23.2°. As for GHA, we're assuming here that we know the equation of time is near zero today. Easy then, right? If that's the case, then the GHA is just the elapsed hours since Greenwich Noon multiplied by 15°! That yields GHA = 150° (for ten hours since noon) + 5° (for twenty minutes) - 8' (taking back 30 seconds), or in other words GHA = 154°52' (yes, you can calculate the Sun's GHA in your head). Now you have Ho, GHA, and Dec. So go on to the next step: suppose your latitude is 40°06' N, what then is the longitude determined by this sight?? One way to proceed: calculate the Sun's hour angle:
   cos(HA) = sin(Alt)/cos(Dec)/cos(Lat) - tan(Dec)/tan(Lat),
and then of course, the hour angle tells you how far the Sun is from your meridian which is identical to the difference in longitude between your vessel and the sub-Sun point. And then you're done! What  did you get? And what longitude do you get if the latitude is 0.1° further north? And if you didn't have a calculator?? How close could you get with a rubber ball?

Frank Reed
Clockwork Mapping / Clockwork Orange (in honor of the Orange Revolution)
Conanicut Island USA

File:

File:

File: