NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
From: Robin Stuart
Date: 2015 Aug 16, 08:49 -0700
Andrés,
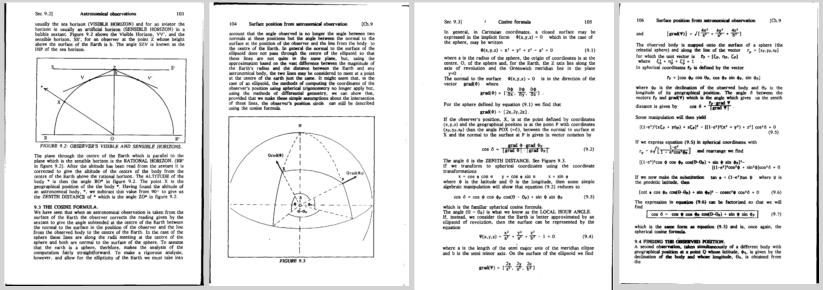
The dot product formula is indeed universal. The (apparent) complication is in the relationship between the direction of the observer's vertical OP on the surface of a sphere vs. an ellipsoid. In the latter case OP does not pass through the centre of the Earth. Your point about thinking of this in terms of 3 dimensional vectors is well taken. I have come to realize that the direction of the vertical for an observer at a given position in geodetic coordinates on the surface of an ellipsoid is by construction parallel to the vertical of an observer with the same coordinates on the surface of a sphere. Williams' demonstration that the form of the cosine rule on the ellipsoid is the same as the cosine rule on the surface of sphere is an immediate consequence of this.
For reference I attach the relevant derivation,
Regards,
Robin